|
|
||||||||
|
|
||||||||
|
Welcome to Norbert Reinecke’s ‘K E Y H O L E’ - O B S E R V A T O R Y |
|
Doppler broadened He I line in Gamma Cassiopeia - A first attempt - |
 |
 |
|
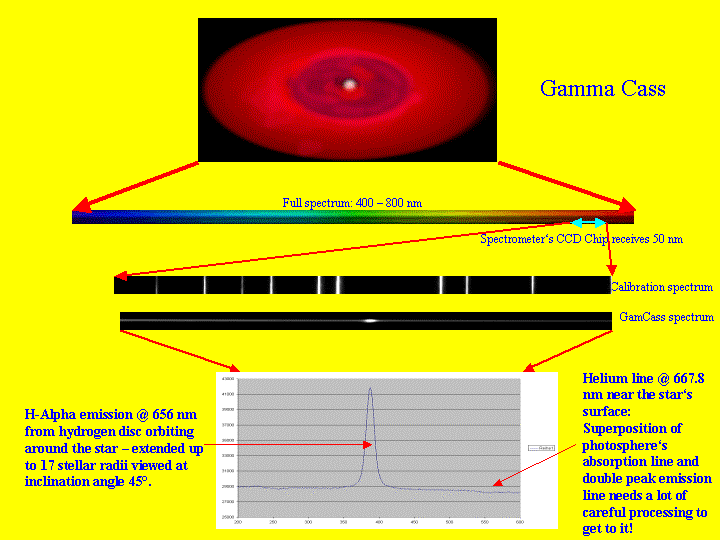
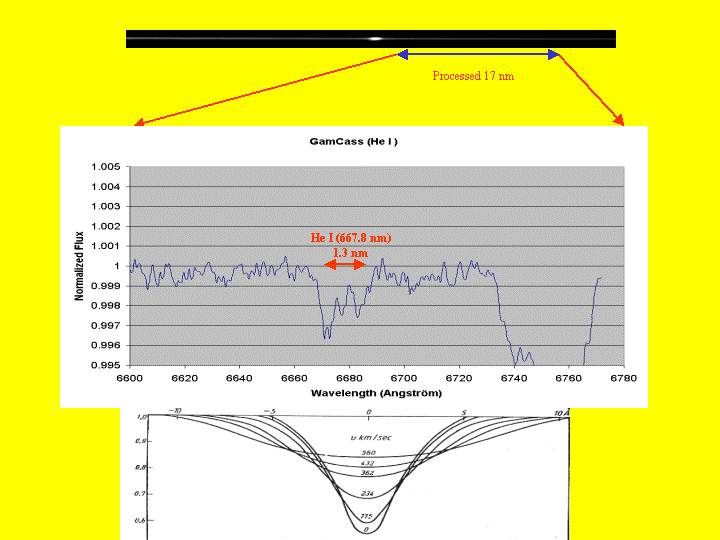
Be stars like Gamma Cassiopeia rotate with extreme speed resulting in the formation of huge gas discs due to the enormous centrifugal forces at their surfaces. The disc is radiating its H-Alpha line in emission at 656 nm. Even more interesting is the photospheric He I emission which is taking place near the star’s surface (1.5 R). At the same wavelength we have additional absorption superimposed onto the emission. The Doppler broadened width of this line at 667.8 nm gives a clue of the speed of the star’s rotation projected onto the LOS to the observer. The measurements depicted above were done under the following conditions: 36 x 60 sec frames without dark or flat field (MX916) as a first test. Above one can follow the steps of generating the result: Wavelength calibration of the received spectrum by means of Ne, accumulation of 36 frames, scanning and processing of the whole spectrum and finally of the interesting part around 667.8 nm. Flux normalization was done with the professional spectral processing program MK32. The Doppler-shift of this spectral line is easily transformed into rotational velocities by means of the following formula: Delta Lamda / Lamda = v / c with v=velocity, c= speed of light, Delta Lamda= measured Doppler shift in wavelength. In this case only half of the maximum Delta Lamda gives us the number we are interested in, since we have to account for a double shift generated by the star’s rotation - simply speaking: one surface moves towards us and the other surface away from us. The depicted 1.3 nm is the absorption line width superimposed on an emission double peak. It has to be divided by 2 which gives us a projected surface speed of approx. 292 km/sec. You can tell from the last graph above that 300 km/sec results in a Doppler line broadening of approximately 10 Angström (= 1 nm) FWHM. Hence, this result seems to make sense having in mind that this is just a first attempt which shall be refined in the near future by applying Darks and Flats to the preprocess. Finally let’s put this result into perspective in terms of stability limits: The limitation to stable rotation of any star is defined by equality of centrifugal and gravitational forces at the star’s equator, i.e. v2 / R = GM/R2 with M being mass and R being radius of the star. A short calculation gives us the stability limit in terms of our sun’s parameters: vrot = 440 sqr (M/Msun / R/Rsun) km/sec
...to be continued soon... |
|
[Home] [Observatory] [Gallery] [Spectroscopy] [Private] |